Knowledge Center > Resources

RF Amplifier Output Voltage, Current, Power, and Impedance Relationship

How much output voltage, current and power can RF amplifiers provide? This question is often asked by novice test engineers as well as seasoned RF professionals. Depending on the application, there is often an underlying desire to maximize one of the three parameters; power, voltage or current. While one would think that a simple application of Ohm’s law is called for, this would only apply given ideal conditions, such as when an RF amplifier with a typical 50 Ω output resistance is driving a 50 Ω load. In this rare case where the load impedance perfectly matches the amplifier output impedance, the power delivered to the load is simply the rated power of the amplifier. There is absolutely no reflected power and thus, there is no need to limit or control the gain of the amplifier to protect it from excessive reflected power.

Unfortunately, such ideal conditions rarely apply in actual “real world” applications. Real amplifiers are required to drive varying load impedances. The mismatch between these “real” loads and the amplifier’s output impedance results in a percentage of the forward power being reflected back to the amplifier. In some cases, excessive reflected power can damage an amplifier, and precautions that may affect forward power are required. Given these realities, how does one go about determining output voltage, current, and power? Again Ohm’s law comes to the rescue, but with the caveat that the actual power delivered to the load (net forward power after the application of any VSWR protection less reflected power) must be determined before applying Ohm’s law. This Application note will highlight some of the major RF amplifier characteristics that impact forward power, as well as net power allowing the use of Ohm’s law, even when conditions are far from ideal.

Back to Basics: Ohm’s Law

Ohm’s law states that the amount of current flowing between two points in an electrical circuit is directly proportional to the voltage impressed across the two points and inversely proportion to the resistance between the points. Thus, the equation I=E/R is the basic form of Ohm’s law where the current I is in units of amperes (A), the Electro-motive Force (EMF) or difference of electrical potential E is in volts (V), and R is the circuit resistance given in ohms (Ω). Applying the standard equation relating electrical power to voltage and current (P=V•A), cross multiplying and rearranging each of the variables results in the equations shown in the Ohm’s law pie chart (see Fig 1) showing the various combinations of the four variables, I, V, Ω, and W. Let’s use Ohm’s pie chart to determine the output voltage, current, and power of a 50 Ω amplifier operating under ideal conditions.

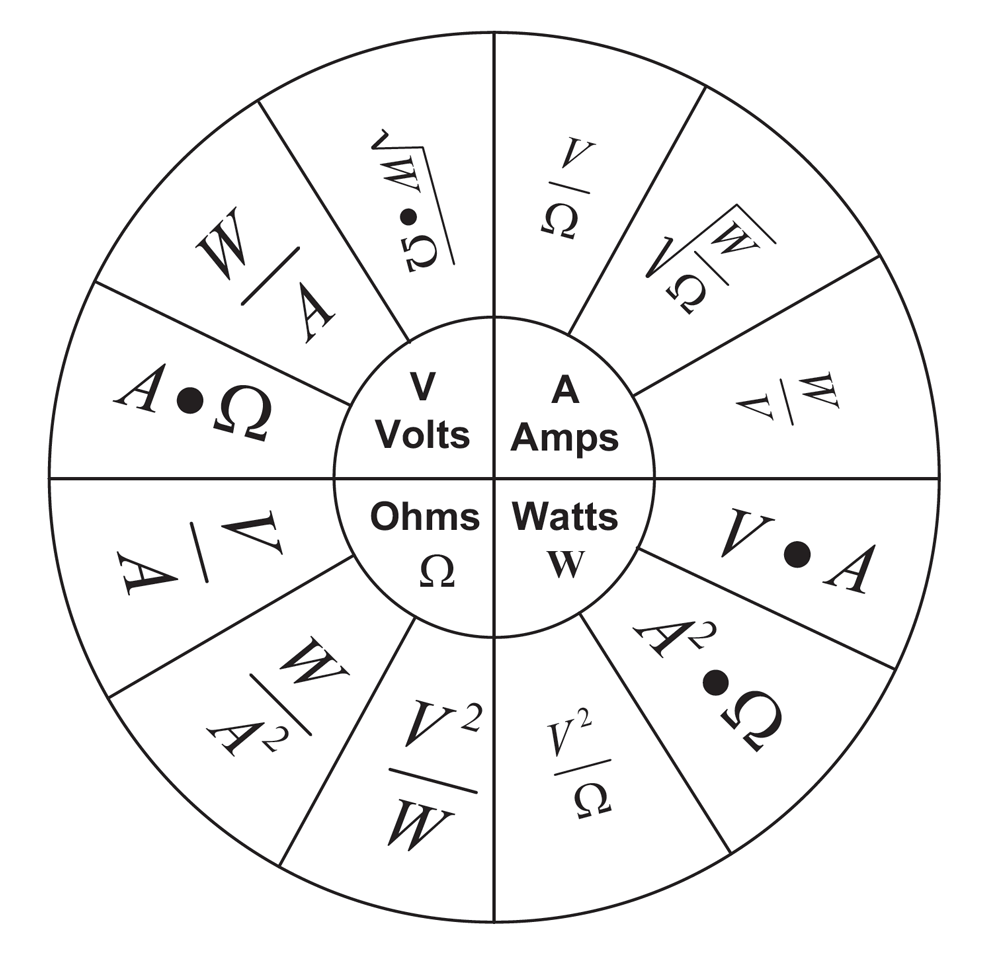
Assume we have a 100W amplifier with 50 Ω output impedance driving a 50 Ω load. This is an ideal situation in that 100% of the forward power will be absorbed in the load and therefore there is no reflected power in this example.

The full 100W will be delivered to the 50 Ω load
Selecting appropriate formulas from the Ohm’s pie chart, one can easily characterize this ideal amplifier.

$$ Volts = \sqrt{Watts * \Omega} $$

Substituting known values:

$$ Volts = \sqrt{100W * 50\Omega} = 70.7 V_{rms} $$

Thus, the output voltage across the 50 Ω load is 70.7 V_rms

$$ Amps = \sqrt{\frac{Watts}{\Omega}} $$

Substituting known values:

$$ Amps = \sqrt{\frac{100W}{50Omega}} = 1.41 A_{rms} $$

The output load current is 1.41 A_rms

As can be seen from the above example, when impedances match, power, voltage, and current are easily determined by the application of Ohm’s law. Now let’s consider “real life” amplifiers and the effects they have on the determination of output voltage, current and power.

Impedance Mismatch: The danger of impedance mismatch and methods used to protect amplifiers

Maximum power is transferred to the load only when the load impedance matches the amplifier’s output impedance. Unfortunately, this is rarely the case. In these “typical” situations, reflections occur at the load and the difference between the forward power and that delivered to the load is reflected back to the amplifier. A voltage standing wave is created by the phase addition and subtraction of the incident and reflected voltage waveforms. Power amplifiers must either be capable of absorbing this reflected power or they must employ some form of protection to prevent damage to the amplifier.

For example, an open or short circuit placed on the 100W power amplifier discussed above would result in an infinite voltage standing wave ratio (VSWR).

$$ \text{Since } VSWR = \frac{Z_0}{Z_L} \text{ for } Z_L>Z_O \text{ and } = \frac{Z_L}{Z_0} \text{ for } Z_L>Z_0 \text{ it can be seen that } VSWR \text{ is always} ≥ 1. $$

With no active VSWR protection, an open circuit at the load would result in a doubling of the output voltage to 141.4Vrms, while a short circuit would increase the output current to 2.82Arms. In either of these worst case scenarios, the 100W power amplifier must tolerate a maximum power of 200W (100W forward + 100W reverse).

Clearly this is cause for concern and amplifier designers must deal with the very real possibility that the amplifier's output might either be accidentally shorted or the load could be removed. Consequently, all amplifiers should employ some form of protection when VSWR approaches dangerous levels. The following is a partial list (most desirable to least desirable) of some methods used:

Overdesign:
- All Solid-state devices and power combiners are conservatively designed to provide sufficient ruggedness and heat dissipation to accommodate infinite VSWR.
- No additional active VSWR protection circuitry is required with this approach.
- This conservative approach is found on AR's low to mid power amplifiers.
Active monitoring of VSWR resulting in a reduction in amplifier gain when VSWR approaches dangerous levels:
- When VSWR exceeds a safe level the forward power is reduced. This technique is sometimes referred to as "gain fold-back" or just "foldback".
- AR's high power solid-state amplifiers will fold-back when reflected power reaches 50% of the rated power corresponding to a VSWR of 6:1and will withstand any amount of mismatch.
Active monitoring of VSWR leading to a shut down when VSWR exceeds a safe level
- This is considered a brute-force technique that can lead to undesirable test disruptions.
- AR does not use this technique in any of its amplifiers.
Active thermal monitoring
- High VSWR will cause a buildup of heat. When a predetermined
- Due to the nature of thermal time constants, this approach is relatively
- AR amplifiers employ some degree of thermal monitoring for circuit
Active monitoring of both output voltage and/or current
- Limits are set for both voltage and/or current similar to restrictions
- If either of the two parameters is exceeded, the amplifier is shut down.

Many amplifiers are designed with little or no concern regarding load mismatch. It is assumed that the application involves a load that matches that of the amplifier. In applications like electromagnetic compatibility (EMC) immunity testing where impedance mismatch is the norm, care must be taken in selecting an amplifier that can tolerate any mismatch while still delivering the required power.

AR solid-state amplifiers have been designed to tolerate extreme load mismatch. They are exceptionally rugged and provide superior protection while delivering the maximum output power to any load. Impedance mismatch is discussed in further detail in “Importance of Mismatch Tolerance for Amplifiers Used in Susceptibility Testing”.

What will be the effect of VSWR protection on forward power, or power available to the load?

Let’s first look at the various methods used to protect AR amplifiers from the ill effects of extreme VSWR.

Class A amplifiers designed to tolerate infinite VSWR: This type of amplifier will not fold-back or shut off when operating into a high VSWR. (Most AR low to medium power amplifiers fit in this category.)
- With these amplifiers, the forward power is always the rated power, and is independent of load
- Example: A 100W amplifier will provide 100W forward power irrespective of load variations
Fold-back based on reflected power: This technique is used for high power AR amplifiers where the reflected power is not allowed to exceed 50% of the rated power.
- These larger amplifiers provide full rated power to the load for any VSWR up to 6:1. As VSWR increases beyond this level, fold-back is used to limit the reflected power to no more than 50% of the rated power, regardless of load variations.
- In this case, available forward power is equal to the rated power until a VSWR of 6:1 is reached. At this point, 50% of the forward power is reflected. For any VSWR greater than 6:1, the forward power is reduced sufficiently to insure that the reverse power never exceeds 50% of the rated power.
- Example: A 1000W amplifier will limit forward power to 50% of the rated power for any load mismatch greater than 6:1. Thus, since 500W is the maximum amount of reflected power, the forward power is 1000W for VSWR ≤ 6:1 and somewhere between 1000 and 500W for VSWR ≥ 6:1.
Voltage and current limited
- For a Voltage/Current limited amplifier, calculations are much simpler. Ohm's law can be directly applied to find net power, voltage, and load current.
  
  The amplifier output impedance is: $$ Z o=\frac{V o l t s_{\text{min } rating}}{A m p s_{\text{min } rating}} $$
- For load impedance higher than the amplifier output impedance the
- For load impedances lower than the amplifier output impedance the amplifier is protected by the current limit. Regardless of how small the load is, the output current will not exceed a value near the specified minimum current rating.
  
  Again applying ohm's law: $$ Watts_{net} = {Amps_{\text{min } rating}}^2 • \Omega_{load} $$ and $$ Volts = Amps_{\text{min } rating} • \Omega_{load} $$

The following comments apply to amplifiers that don’t use one of the AR style VSWR protection methods listed above:

Amplifiers that protect by shutting down or turning off the RF output:
- Forward power will be 0 if VSWR is excessive. This may occur at a VSWR as low as 2:1, but more often occurs for a VSWR somewhere between 2:1 and 3:1.

Clearly, amplifiers that either don’t employ VSWR protection or use this brute force VSWR scheme cannot be used in applications where load mismatches are expected. Amplifiers that employ fold-back schemes at even lower VSWR levels than noted above are also in this category and are unsuitable for applications characterized by high load VSWR such as EMC immunity testing and research applications where load impedance is unknown.

Output power loss due to load mismatch

We have concentrated on the topic of forward power up to this point. This is the power actually available at the load. Jacobi’s Law, also known as the “maximum power theorem” states that “Maximum power is transferred when the internal resistance of the source equals the resistance of the load, when the external resistance can be varied, and the internal resistance is constant.” This effect is clearly observed when load impedance differs (greater or less) from the amplifier’s output impedance. As VSWR increases, an ever greater portion of the forward power is reflected back to the amplifier. Since net power is calculated by subtracting the reflected power from the forward power, it is apparent that any VSWR other than 1:1 will reduce the actual power absorbed by the load.

The amount of power delivered to the load can be calculated using the following standard RF formulas:

Reflection Coefficient:

$$ r = \Bigg | \frac{Z_2-Z_1}{Z_2+Z_1} \Bigg | $$

,where the two impedances are the load impedance and the output impedance of the amplifier.

Once the forward power has been determined and the reflection coefficient calculated, the net power delivered to the load is found by merely substituting values into the following equation:

$$ Watts_{Net} = 10^{\Bigg(\frac{10•Log\big(1-r^2\big)=10•Log(Watts_{FWD})}{10}\Bigg)} $$

Furthermore, given the net power and load impedance one can then calculate the output current and voltage using Ohms law.

Real Examples

Now that we have investigated the nuances involved in determining output power, voltage, and current of RF power amplifiers in general, let’s look at four existing AR amplifiers and how they deal with load mismatch.

Example 1: Most low and medium power amplifiers are of the Class A design and have nominal 50 Ω output impedance. A typical amplifier of this type is the 75A400 power amplifier:

10 kHz – 400 MHz bandwidth
75W minimum RF output
No active protection is required given its very robust, conservative design
Full forward power is provided into any load impedance

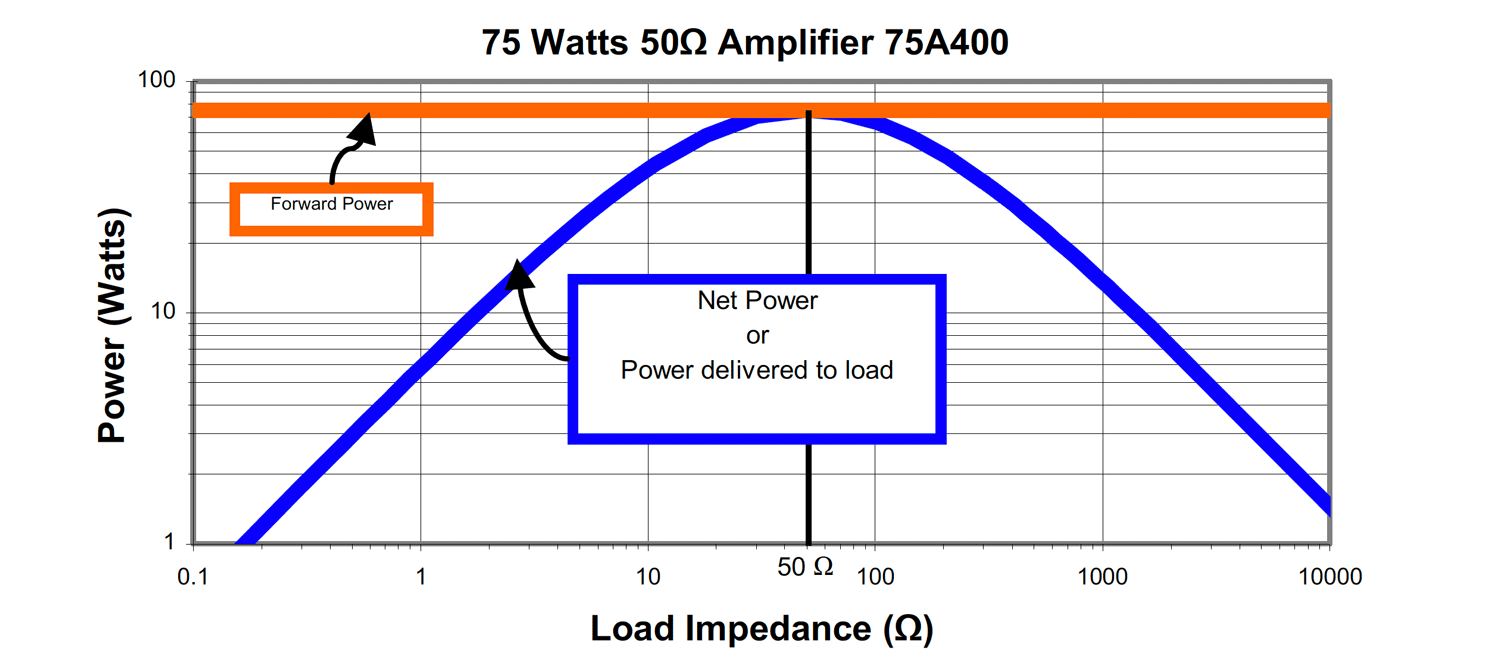
Figure 2 clearly demonstrates the best possible scenario provided by the 75A400. The forward power is constant at 75W irrespective of load impedance. The center point of the graph demonstrates maximum power transfer per Jacobi’s Law where the 50 Ω amplifier is driving a 50 Ω load and the blue output power curve clearly demonstrates the reduction in net power per the maximum power theorem as the load varies from the ideal of 50 Ω. Note that even though 75W is available independent of the load impedance (orange curve), there is only one point where the power delivered to the load is equal to the forward power; the point where the load impedance matches the amplifiers output impedance. The fall-off of the power delivered to the load on either side of the 50 Ω load impedance is the result of load VSWR causing an ever increasing portion of the forward power to be reflected back to the amplifier. Recall that

$$ P_{n e t} = P_{f w d} - P_{r e f} $$

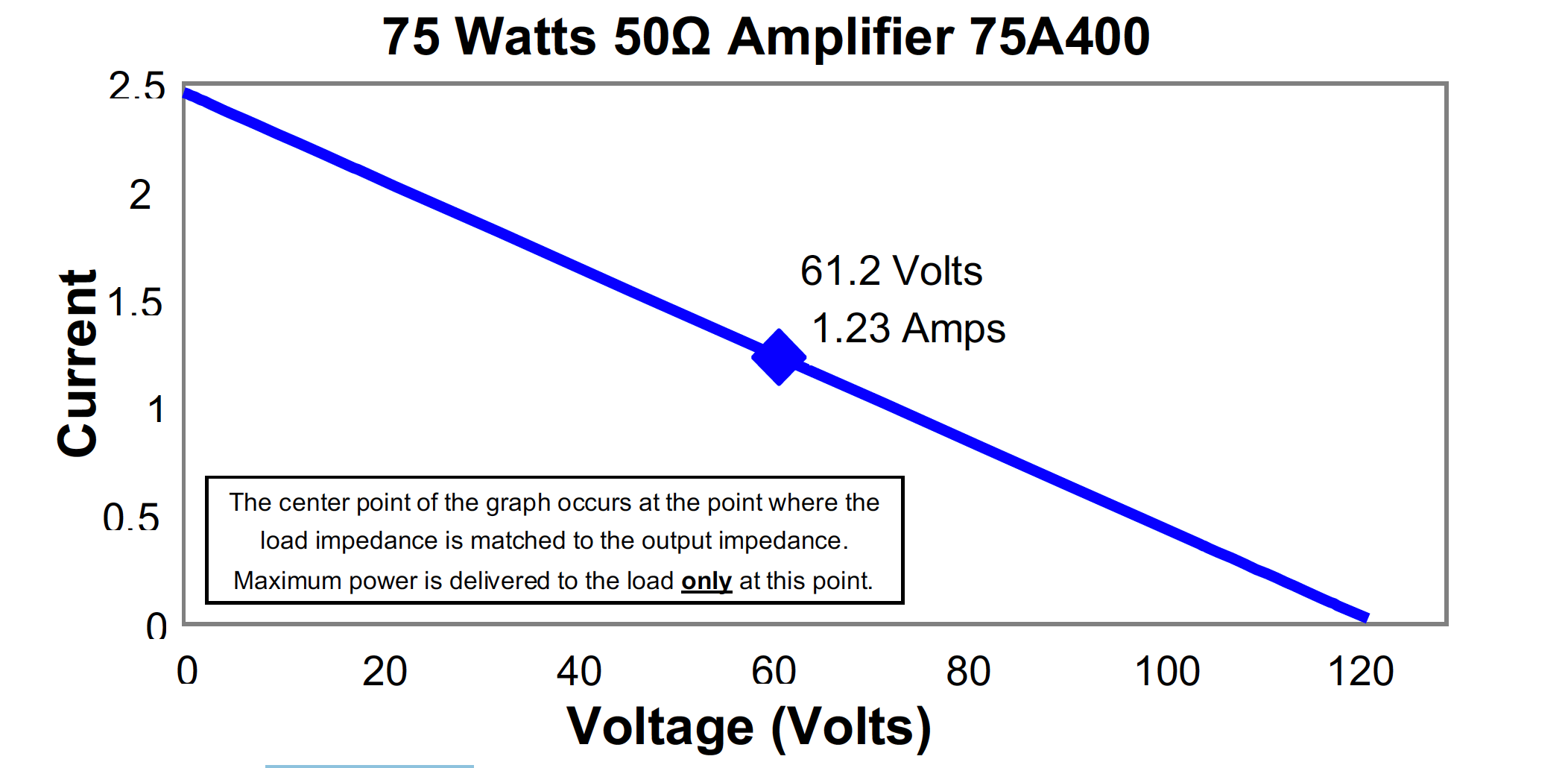
Figure 3 plots the voltage and current over the entire range of load impedance. The center point represents the voltage and current produced when the load impedance matches the amplifiers 50 Ω output impedance. Loads greater than 50 Ω are plotted to the right of the center point and loads less than 50 Ω appear to the left. The endpoints demonstrate the two possibilities of a worst-case mismatch; an open where the output voltage is at a maximum with zero current, and a short where the current is maximum with zero voltage.

The above graphs are based on the minimum rated output of the amplifier across its entire operating frequency range. There most likely will be spotted within the frequency range where the output power will exceed the specified minimum rated output power. To avoid unexpected results, always request a copy of specific production test data before placing an amplifier in service.

Example 2: High Power Solid-State amplifiers by necessity employ active VSWR protection. Take, for example the 1000W1000D.

80MHz – 1000MHz bandwidth
1000W minimum RF output delivered into a 50 Ω output impedance
Active protection kicks in to reduce the gain when reverse power is measured at 500W; this is a VSWR of 6:1 when using the amplifier at rated power.
This fold-back protection limits the reflected power to 500W maximum

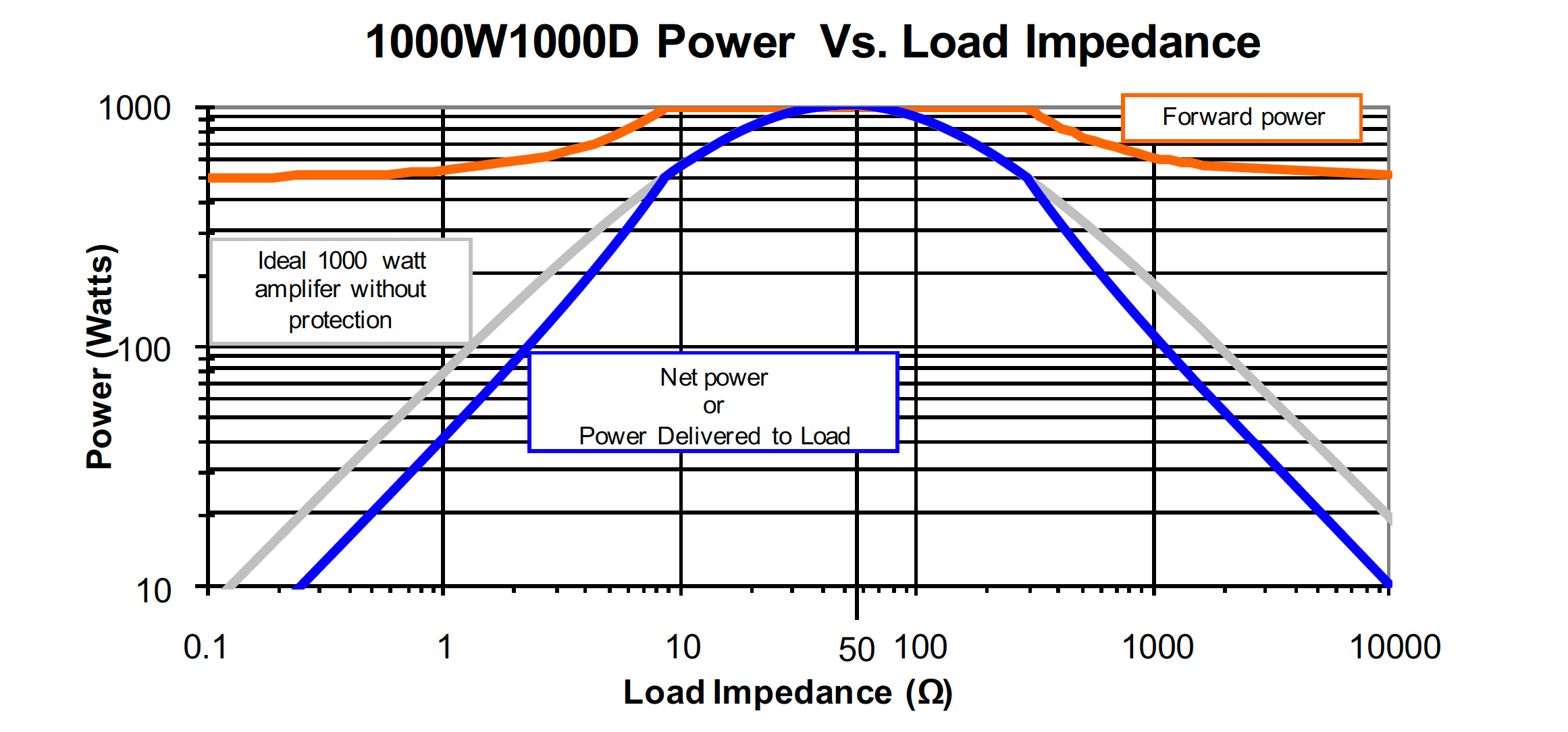
The 1000W1000D is an example of one of AR’s high power amplifiers that folds back when reverse power reaches 50% of rated power. Even though the amplifier does fold back, a considerable amount of power is still being delivered to the load. In many cases, other manufacturers of high-power amplifiers would not be able to handle such conditions, and forward power would either be shut down completely or reduced drastically.

In power critical applications, an impedance matching transformer similar to the one used in the AR 800A3A could be used to match the amplifier to the load. However, since matching transformers tend to be narrowband, this approach may prove impractical if the 1000W1000D were to be operated over its entire frequency band. In this case, a series of narrow-band transformers could be switched into the application as the frequency dictated or simpler yet, the user could opt for a higher power amplifier.

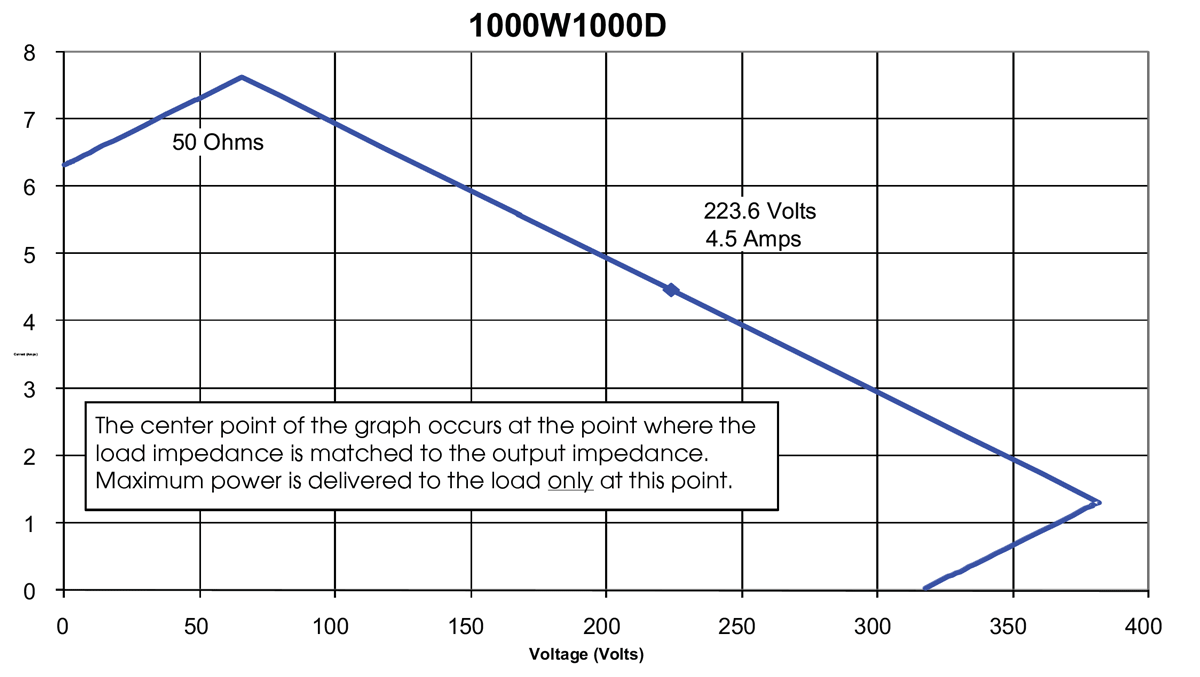
The above graph demonstrates that even though fold-back occurs at a VSWR of approximately 6:1, significant output voltage and current are still delivered to the load.

Example 3: Much has been said so far regarding the importance of impedance matching. The 800A3A is an example of a unique amplifier that provides the user with selectable output impedance to match a wide variety of applications.

10 kHz – 3MHz bandwidth
800W minimum output power rating
An internal user-selectable impedance transformer provides 12.5, 25, 50, 100, 150,
Active protection kicks in when VSWR exceeds 6:1 to reduce the gain
This fold-back protection limits the reflected power to 400W maximum

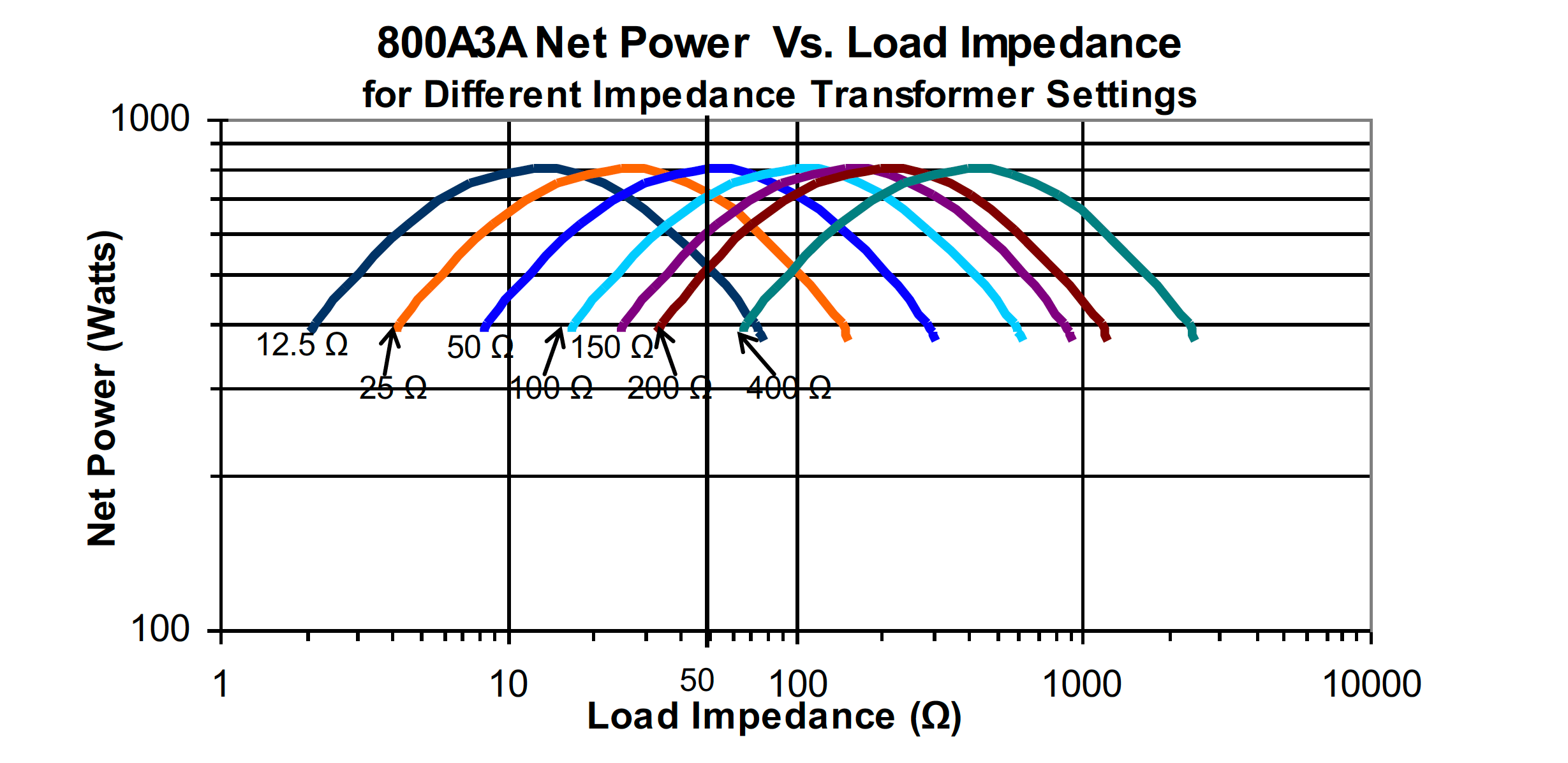
The internal impedance transformer of the 800A3A allows this amplifier to have output impedance that matches that characterized by a variety of applications. External transformers are available to extend the usefulness of the 800A3A to include even more applications.

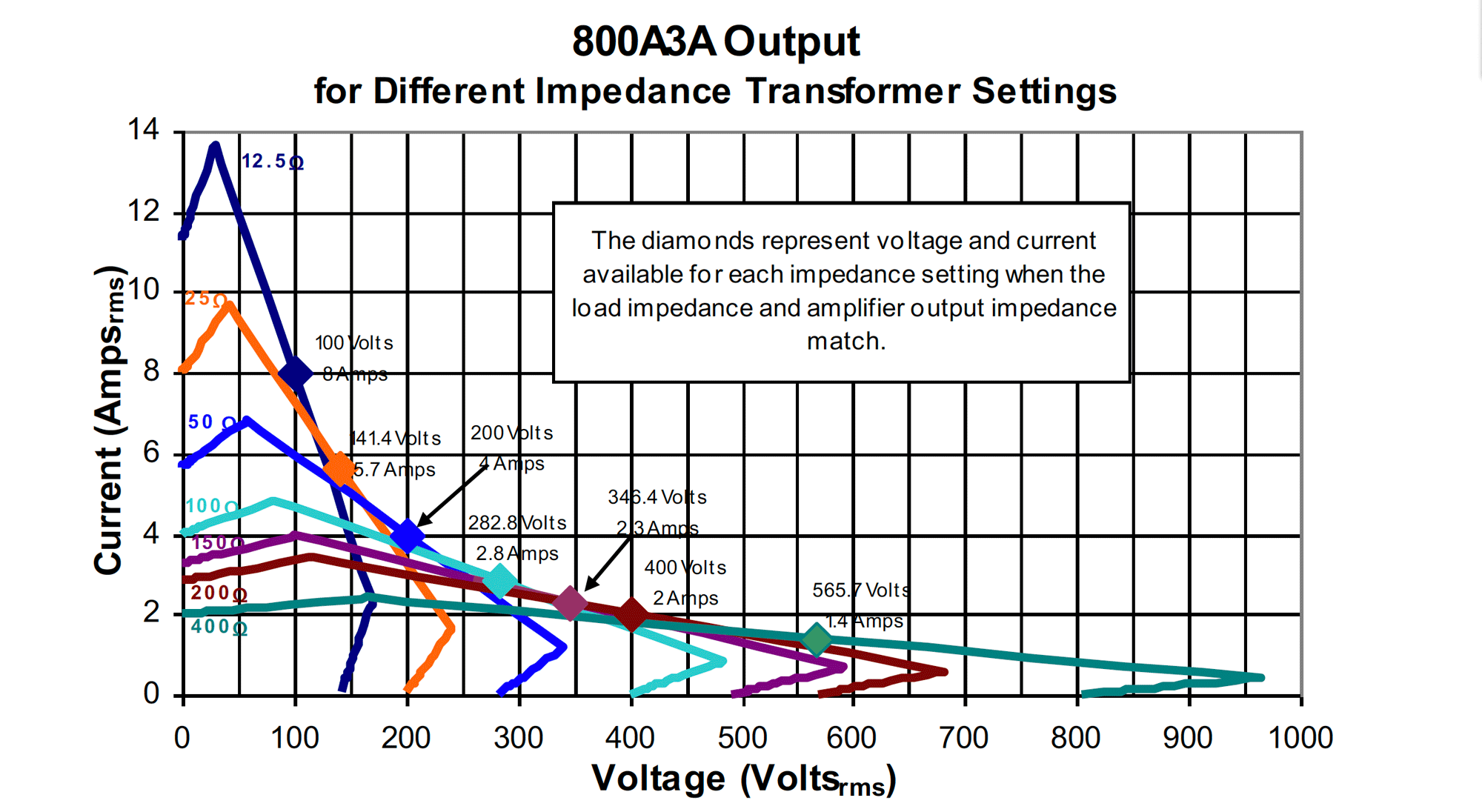
Figure 7 clearly highlights the benefits of an amplifier with an internal impedance matching transformer that facilitates a better match with varying loads. The range of output voltage and current is considerably greater than what is provided by a standard 50 Ω amplifier.

Example 4: The salient characteristics of high power, broadband, and very low output impedance (typically <1 Ω) of the 350AH1 uniquely appeal to low-frequency applications. The 350AH1 differs from other amplifiers in this class in that it is a full sized bench-top instrument with “real-time” graphical color displays of output voltage and current. Its extremely robust design ensures that it can stand up to the most demanding applications.

10 Hz – 1 MHz bandwidth
The minimum rated output power is 350W into a 1.8 Ω load. This equates to a minimum of 25 volts and 14 amps into 1.8 Ω. (Power de-rated above 300 kHz)
Source impedance is rated at <1 Ω (Since the output voltage and current are
Effective source impedance is 1.8 Ω (Zo=Vo/Io= 25V/14A)
Output protection limits both the voltage and current at rated values into any load. For loads less than 1.8 Ω, the output current is limited. For loads exceeding 1.8 Ω the output voltage is limited.

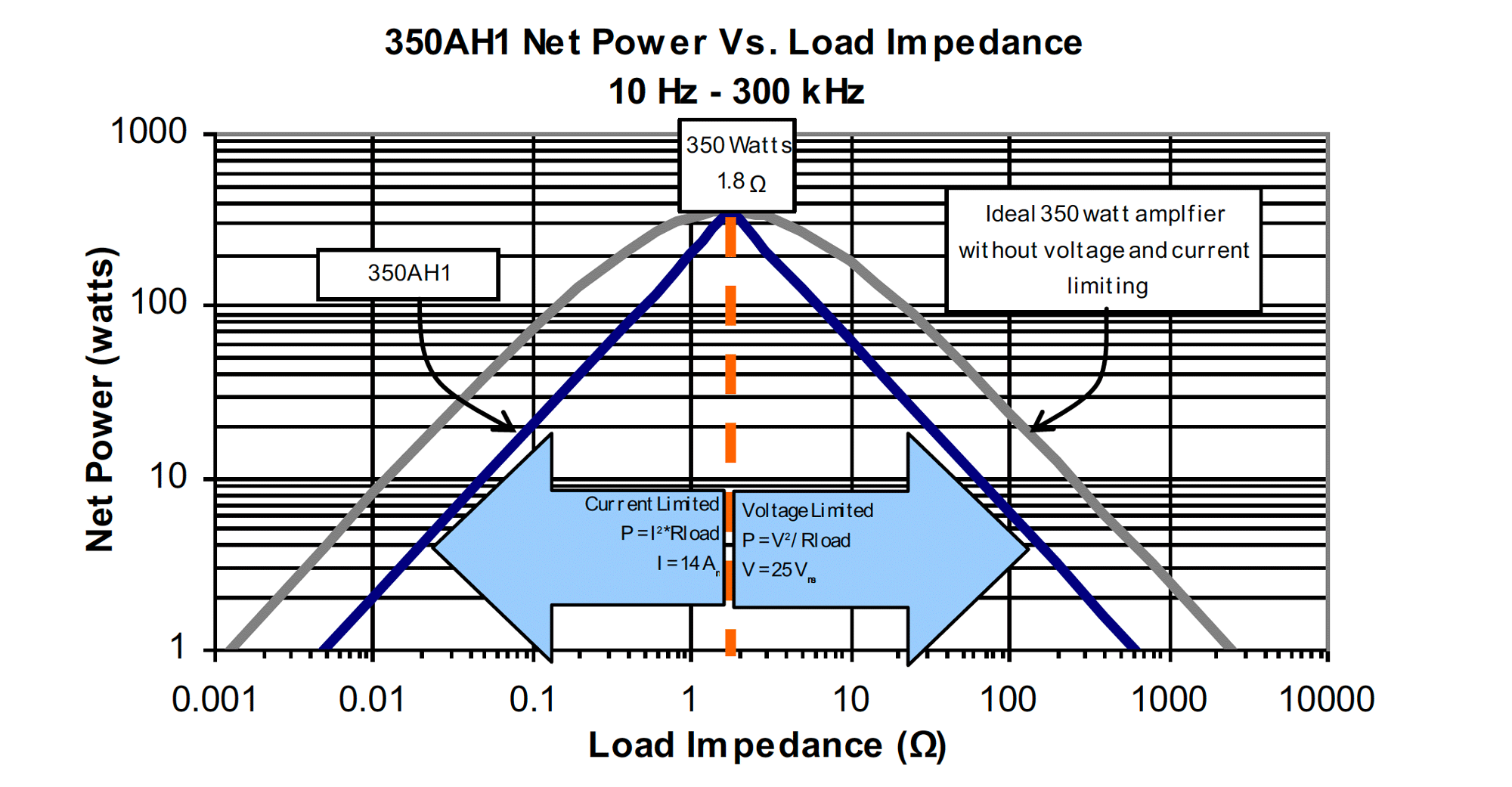
This is an example of an amplifier with a 1.8 Ω effective output impedance. Due to the voltage and current limiting protection of the amplifier, VSWR does not play a role in lost power delivered to the load.

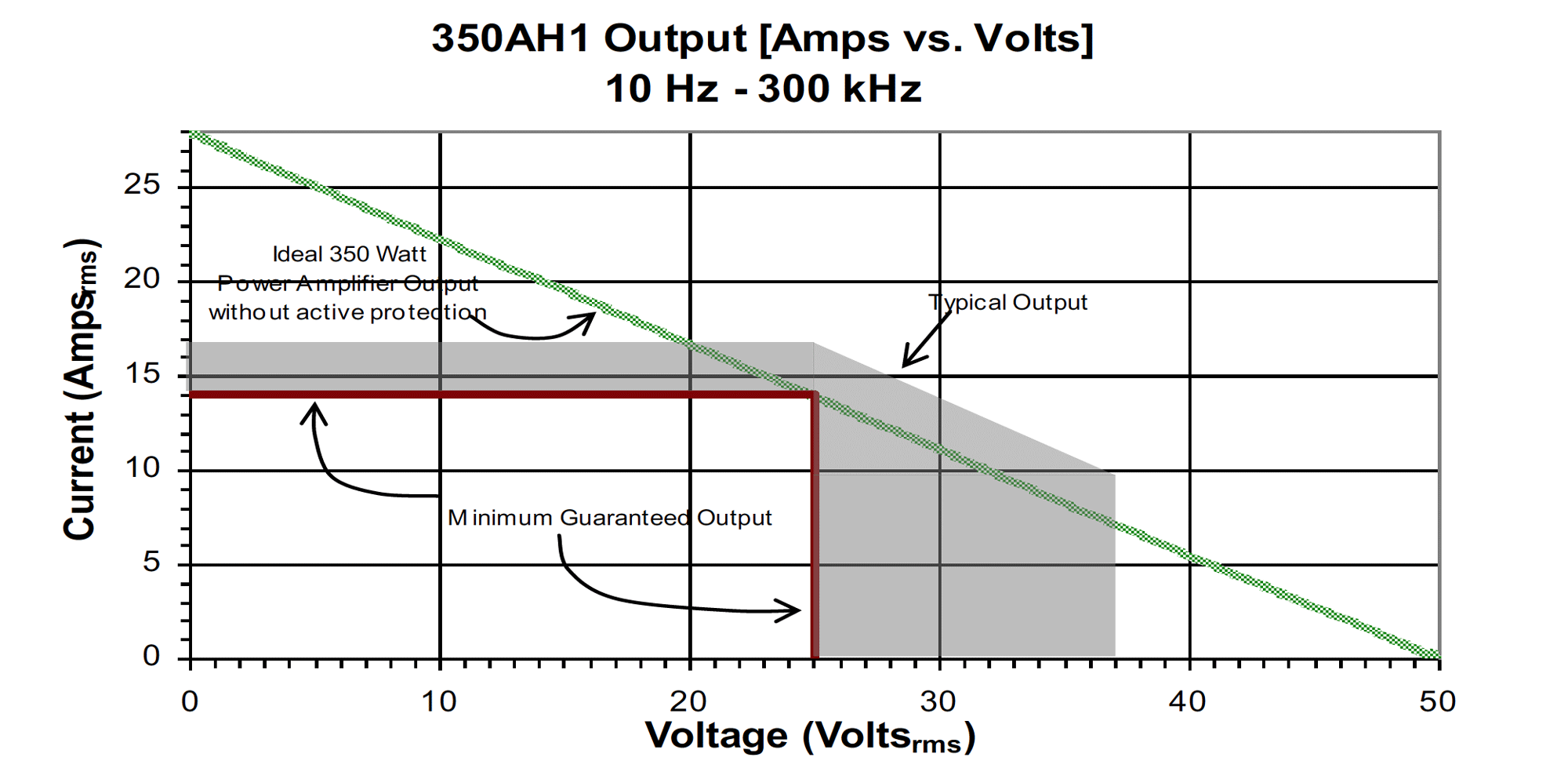
Figure 9 plots the available output voltage and current from the 350AH1. The gray area is provided to indicate a more “typical” output profile. Individual amplifier characteristics will vary and are somewhat influenced by operating frequency and system losses.

Summary

The age-old question of “How much output voltage, current, and power can I expect from my amplifier?” can in rare cases be answered by merely applying Ohm’s law assuming the net power or power delivered to the load is simply the rated power output of the amplifier. In most cases, practical issues such as VSWR and forward power concerns must be considered before applying Ohm’s law. While this application note has provided guidance in this matter, AR firmly believes that the best approach is to apply actual test data when calculating output parameters. If you are the least bit uncomfortable with this exercise, feel free to contact one of our Application Engineers. We would be more than happy to guide you through the process.